synopsis: (1) statistical uncertainty increase due to reset level variations within a staring measurement at a constant flux level. The statistical uncertainty is probably small: less than 2% unless the measurement is very long. (2) residual systematic photometric uncertainty for multi-filter and multi-aperture staring observations especially with detectors P3, C100 and C200. The residual systematic uncertainty after correction is estimated to be at most 10%. (3) residual systematic photometric uncertainty for measurements in which the signals cover a high dynamic range such as in maps with bright features and in chopped observations. The estimated systematic uncertainty is at most 10%. (4) systematic photometric uncertainty in PHT-S observations. Since the integration ramps for PHT-S observations are not linearized the residual systematic error is probably better than 20%.
limitations and applicability:
(1) no correction applied for PHT-S (2) strong signals with low bias
detectors are not properly corrected.
description:
Removal of systematic non-linearities (``kinks'') in integration ramps by
adding to each read-out voltage a positive or negative voltage in order
to obtain a linear or ``straight'' integration ramp. It is assumed that
the deviation from non-linearity only depends on the read-out voltage level.
This correction is applied to all detectors except PHT-S.
purpose correction:
The integration ramps are not straight but show systematic kinks and
curvatures. The non-linearities cause systematically different slopes (signal
level) for different parts of the ramp making the ramp signal an ill-defined
parameter and a sensitive function of the voltage range covered by a ramp.
The correction is based on the assumption that the deviation from linearity
only depends on the CRE voltage level of a read-out.
Consequently, a ``straight'' ramp is derived by adding a voltage correction
to each read-out. The corrections were obtained from an empirical integration
ramp constructed from averaging many representative integration ramps.
The deviations of this average ramp from a generic straight ramp yield the
correction terms (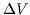
)
as a function of read-out voltage.
For the low bias-detectors (P3, C100 and C200) the non-linearity is not
only a function of CRE voltage, but also a function of the detector
photo-current. The charge accumulated on the integration ramp feeds
back and reduces the bias voltage ("debiasing"). This effect depends
on the voltage difference due to the charges above the reset voltage
level. The stronger the photo-current, the bigger the downward
curvature of the integration ramps. No specific correction is made for this
effect. It should be noted that also all calibration observations were
linearized with the same correction tables.
uncertainty/noise introduced:
In case the reset level changes within a measurement, the uncertainties
in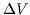
widens the signal distribution. Since the reset level
changes only slowly in time for measurements of the same signal
strength the contribution to the statistical uncertainty is small,
less than a few percent is estimated. However, the reset level changes
when the signal level varies. This can happen in obsevations using
multi-filter, multi-aperture, chopped or mapping modes.
For PHT-S which is not corrected at all, and for P3, C100 and C200 which are low bias detectors, the ramp non-linearity is not removed completely. Since different signal strengths require different corrections, the residual error may cause systematic uncertainties depending on the signal strength.
auxillary data:
Cal-G files: PPCRELIN, PC1CRELIN, and PC2CRELIN. These files also
contain the statistical uncertainties of the corrections.
keyword(s):
none