



Next: THE COOKBOOK
Up: ISOPHOT OBSERVER'S MANUAL
Previous: Sensitivity Limits at
The absolute photometric accuracy to be achieved with ISOPHOT ultimately
depends upon the accuracy of the celestial standards. There are
several calibration programmes from the ground and from the KAO aimed at
establishing a network of primary and secondary standards. For wavelengths
less than 50 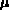 m, stars will be the primary standards. At far infrared
wavelengths, asteroids and planets are primary calibrators.
As secondary standards, planetary nebulae will be used.
The goal is to establish the fluxes of these objects to an accuracy of
10 to 20%.
m, stars will be the primary standards. At far infrared
wavelengths, asteroids and planets are primary calibrators.
As secondary standards, planetary nebulae will be used.
The goal is to establish the fluxes of these objects to an accuracy of
10 to 20%.
All ISOPHOT single-pointing measurements involve an FCS calibration for each
detector used. Each map or scan requires two FCS calibrations both at the
beginning and the end of the measurement. The S/N of the internal calibration
measurement depends on the requested S/N of the astronomical
measurement,
e.g. high calibration accuracy will not be sought when the source is only
marginally detected. The internal calibrations will serve as transfer measurements
to the celestial photometric standards.
Different absolute detector responsivities, perhaps due to e.g. a
different environment in irradiation by high energy particles on various orbit
positions can be removed in this way.
One significant factor for the relative accuracy - the reproducibility of a
certain signal level - has been described in Section 2.8
and some guidelines for optimising the accuracy have been given. It can be
assumed that the FCS calibrations reach, in general, the same level of accuracy
as the astronomical measurement so
that relative photometric accuracies with
respect to sky standards of about 5% could be achieved at best.
Furthermore, the determination of the sky background is essential for accurate
photometry of fainter sources. Background gradients or background structure can
strongly influence the flux level of the object signal. In particular, the
cirrus confusion
at wavelengths beyond 50 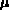 m should be carefully considered
(see ISO Observer's Manual for details). It is advised
to measure several background fields either by triangular or sawtooth
chopping or by sparse mapping. The most accurate procedure but also the most
expensive in terms of observing time is mapping.
m should be carefully considered
(see ISO Observer's Manual for details). It is advised
to measure several background fields either by triangular or sawtooth
chopping or by sparse mapping. The most accurate procedure but also the most
expensive in terms of observing time is mapping.
For standard single-pointing photometry of point sources a
correction factor must be applied to correct for truncation of the point spread
function by the aperture field of view.
Photometry of extended sources will yield somewhat worse accuracy than
for point sources. Beside the uncertainties listed above the flatness of
the beam profile, which is known to about 5%, is another factor affecting the
accuracy.
The design of the PHT AOTs already makes some provisions to improve the
photometric accuracy of certain observing modes:
-
Chopping should be used for faint sources to cancel out slow detector
baseline drifts.
-
The PHT-C mapping AOT uses the chopper for differential measurements
in order to assess the baseline drift, a feature which is not possible
with a simple repetition of staring mode observations.
-
The PHT-C mapping AOTs use sampling patterns in which each sky position of a
map is viewed by all pixels of the array in order to optimise the flatfield correction.
-
Polarimetric observations are very sensitive differential measurements
with different polariser settings, so each observation includes
non-polarised photometry
for consistency checks. For very long integration times per polariser, the
time is divided into several blocks during which all three polariser
directions are set up. These time blocks, which are at least 64 seconds long,
are long enough to permit assessment of short term drifts due to flux changes
introduced by
the wheel rotations but still short enough to cancel out any long-term
drift. The ability to measure polarisation levels below 3% may be
improved if the measurement is repeated some time later (a few months)
when the orientation of the focal plane has rotated relative to the sky;
such differential methods can be used to determine the instrumental
polarisation to high accuracy. The repetition of observations may be
limited, however, by visibility restrictions due to spacecraft
orientations relative to the Sun (see ISO OBSERVER'S MANUAL).
-
Measurements of absolute sky brightness can be improved in accuracy by using
the PHT internal fine calibration sources as reference.
-
The dark current of a detector can be determined by measuring against the cold,
i.e. switched off, fine calibration source.




Next: THE COOKBOOK
Up: ISOPHOT OBSERVER'S MANUAL
Previous: Sensitivity Limits at
Tue Dec 6 17:24:45 MET 1994
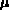 m, stars will be the primary standards. At far infrared
wavelengths, asteroids and planets are primary calibrators.
As secondary standards, planetary nebulae will be used.
The goal is to establish the fluxes of these objects to an accuracy of
10 to 20%.
m, stars will be the primary standards. At far infrared
wavelengths, asteroids and planets are primary calibrators.
As secondary standards, planetary nebulae will be used.
The goal is to establish the fluxes of these objects to an accuracy of
10 to 20%.



