



Next: 6. Data processing
Up: ISOLWS DATA USERS MANUAL
Previous: 4. Instrument Characteristics
Subsections
5. Calibration and Accuracy
5.1 Calibration philosophy
The calibration of the LWS instrument can be split into two main areas:
the instrumental characteristics and the astronomical calibration.
In this context, the instrumental characteristics calibration are those
properties of the instrument which need to be known in order to extract
the astronomical information from the data stream. These are mainly used
in Derive-SPD (cf. section 6.3).
Astronomical calibration, on the other hand, is needed to convert from the
internal "engineering" units of the instrument to astronomical units. This
is mainly used in Auto-Analysis (cf. section 6.4).
The instrumental characteristics calibration can be divided into:
- Detector characteristics
- Electronic characteristics
- Fabry Perot characteristics
- Optical characteristics
The astronomical calibration includes, for both grating and Fabry-Perots:
- Wavelength calibration.
- Relative spectral response and flux calibration
5.2 Instrument characterisation procedure
5.2.1 Detector characteristics
- Debiasing parameters
- These are measured with the
internal illuminators, using different compensation voltages at the
start of the ramps. The compensation levels induce different
effective bias levels for the ramps. From the second order polynomial
fits to the data the debiasing parameter a (see section 4.2)
can be determined.
- Detector Dark Current
- The dark currents can only be
measured together with any current induced by background or stray
radiation. To minimize this background radiation
the measurement is done by placing a Fabry-Perot - deliberately made
non-parallel - in the beam. Tests have shown that
this gives the lowest background on the detectors, the FP acting as a
highly efficient blocking filter. ISO is then pointed to a
relatively clear part of the sky to get minimal straylight from
off-axis sources. The observation
is done with several bias levels. The grating is scanned to look for
spectral signatures in the dark current, and the illuminators are used
to check for responsivity changes during the dark current measurement.
5.2.2 Electronics characteristics
No need for in-orbit characterisation could be identified. All characteristics
are determined in the laboratory and while building the instrument. The
important characteristics are:
- The time constants of the high pass electronic filters
- The gains of the amplifiers
During the Spacecraft Commissioning Phase (SCP) several tests were
done to detect possible large changes in these values that would
point to problems with the instrument.
5.2.3 Fabry Perot characteristics
The optimum offsets for the
Fabry-Perot etalons for FPS and FPL are determined by observing an unresolved
line at several offset values around the
expected optimum values (offset mapping). This gives a set of line
spectra. For each of these spectra the FWHM of the line and the peak heights
are determined. From the contour maps of the FWHM of the line versus the
two FP offset values (for the coils of one FP) the position of the minimum
FWHM is determined. The offset values corresponding to this minimum
are optimum for parallelism.
5.2.4 Optical characteristics
The beam pattern of the instrument is determined by taking full grating spectra
over a raster map of a strong source. The result is a map of the beam-pattern
of the instrument as a function of wavelength.
5.3 Astronomical Calibration procedure
5.3.1 Wavelength calibration
- Grating mode calibration
-
This is done using first a full grating scan on a source with known lines
to identify the lines suitable for wavelength calibration, those that are
strong enough to give good signal-to-noise and that are narrow enough
to determine an accurate line centre. Individual scans of these lines
are then made to provide accurate line positions. These line positions
are fitted to the known wavelengths with a third order polynomial to
give a grating calibration that is adequate for the low resolution mode
of the instrument.
- Spectral element
-
The size of the grating spectral element is determined using the
mixed mode observations. Detailed FP scans are made with the grating
at a fixed position, giving a very detailed profile of the grating
response function: from this, the size of the spectral element
(Full Width Half Maximum) is determined. The observation is done
for several detectors and grating positions to get the wavelength
dependence of the profile and spectral element.
- Wavelength Calibration
-
The wavelength calibration of the Fabry-Perots is carried out by observing
strong, narrow lines with known wavelengths. The centres of these
lines are determined as a function of the commanded position of the
Fabry-Perot. The corresponding order of interference and etalon gap are
calculated abd the gap is fitted as a third order polynomial in
commanded position.
- Spectral element
-
The size of the Fabry-Perot spectral element should be determined using the
wavelength calibration observations. The Fabry-Perots are scanned over
lines known to be narrow, on sources with a reasonably well known
velocity dispersion. The Fabry-Perot profile is determined by deconvolving
the observed lines shape with the expected line shape given the source
structure and velocity dispersion. From this
the size of the spectral element (Full Width Half Maximum) is
determined. The observation is done for several lines to get the wavelength
dependence of the profile and spectral element. Due to the lack of
suitable sources for this calibration. Even the source G0.6-0.6, which has
very narrow lines has proven to give difficulties for the determination
of the FP spectral element, since the velocity dispersion of the source
is close to the resolution of the FPs. Investigations are still ongoing
to improve the methods for obtaining the FP resolution from data that has
been taken in orbit (instead of in the lab with laser lines).
5.3.2 Relative Response and Flux calibration
The absolute flux calibration is done by
directly comparing the detector photo current of a standard source
with the known (model) flux (corrected for the optical characteristics of the
telescope and the LWS instrument).
The LWS has been calibrated by observing Uranus, a source for which a good
model exists and which is point-like in the LWS beam. At each wavelength
the expected flux density (power per unit area) from Uranus, within the
spectral resolution element, is calculated from the model and the
corresponding photocurrent is measured. This calibration therefore
provides a direct relationship - for a point source - between the
photocurrent and the flux density at the entrance pupil of the ISO
telescope. This relationship is then used to assign a flux density to
the observed photocurrent from any other source.
The observations for the flux calibration are repeated for several
bias levels.
The grating relative response needs to be known before the FP relative
response calibration and the FP transmission calibration can be performed.
For the photometric calibration and the relative response calibration
several sources were used during PV phase and during the routine
calibration observations. Table 5.1 gives the sources
for the different calibrations.
Table 5.1:
Sources used for
the photometric calibration and the relative response calibration
of the LWS grating and Fabry Perot subsystems. The primary source for
the grating flux calibration is Uranus, the other sources are observed
regularly for monitoring purposes.
| Source |
Type |
Observation |
| Absolute Flux Calibration and monitoring |
| Uranus |
planet |
Fixed grating position On and Off source and full scan |
| Neptune |
planet |
Fixed grating position On and Off source and full scan |
| Ceres |
astroid |
Fixed grating position On and Off source |
| Pallas |
astroid |
Fixed grating position On and Off source |
| Vesta |
astroid |
Fixed grating position On and Off source |
| Arcturus |
star |
Fixed grating position On and Off source |
| Aldebaran |
star |
Fixed grating position On and Off source |
 Dra
Dra |
star |
Fixed grating position On and Off source |
| S106 |
HII region |
fixed grating and full scan |
| G298.288 |
HII region |
fixed grating and full scan |
| NGC6543 |
PN |
full grating scan |
| NGC7027 |
PN |
full grating scan |
| Relative response calibration |
| Uranus |
planet |
End to end grating scans (extended range) |
| FP Relative Response and transmission Calibration |
| Jupiter |
planet |
FP scans with fixed grating (mixed mode) |
|
For the Fabry Perot response the FP is scanned with a high oversampling
with the grating at a fixed position (mixed mode observations). Again here
the source spectrum is
removed (by means of a model or a grating scan). Finally the profile
is normalized to the absolute transmission at a given wavelength (the
FP transmission is determined in a separate procedure, see below).
The FP transmission is determined by using scans of strong lines with
both the grating and the Fabry Perot. Comparing the output of the
detectors for these scans of the same line for grating and Fabry Perot
gives the the Fabry Perot transmission at the wavelengths of the lines.
5.4 Calibration sources and achieved accuracy
5.4.1 Spectroscopic calibration
The wavelength calibration of the LWS grating consists of the set of
detector angles and the coefficients of the third order polynomial fit
of the LVDT value versus grating angle. Since version 6 of the OLP pipeline,
the detector angles given in Table 5.2 are used. The shifts in this
table indicate the change of the angles with respect to the ones determined
on the ground. The angles have not changed in version 7 of the OLP pipline.
Table 5.2:
The detector angles derived from the grating
wavelength calibration, and the difference between the in orbit and
ground based angles for each of the ten detectors.
| det. |
SW1 |
SW2 |
SW3 |
SW4 |
SW5 |
LW1 |
LW2 |
LW3 |
LW4 |
LW5 |
| angle |
67.80 |
58.74 |
49.71 |
40.73 |
31.72 |
63.26 |
54.29 |
45.27 |
36.275 |
27.32 |
| shift |
-0.1 |
-0.01 |
0.0 |
0.0 |
+0.02 |
+0.02 |
+0.01 |
+0.01 |
+0.04 |
+0.04 |
|
During the routine checks of the grating wavelength calibration it was found
that a change in the calibration occurred in revolution 346. Therefore it was
decided to use a timedependent wavelength calibration consisting of
two sets of parameters, one valid for observations performed before
revolution 346 and one for observations performed in revolution 346 and
after. The calibration was derived using 7 fine structure lines observed with
at least two detectors each in a number of different sources (mainly
planetary nebulae and HII regions). Table 5.3 gives an overview
of the lines and sources that were used for the calibration.
Table 5.3:
The sources
and emission lines that are used for the wavelength
calibration of the grating and of both FPs. For the FPS calibration all
lines were observed in three FP orders. For the FPL the lines were
observed in two orders. Type indicates the source
type (PPN: Proto Planetary Nebula; PN: Planetary Nebula).
| Source |
Type |
Lines |
| Grating wavelength calibration |
| NGC6543 |
PN |
52, 57, 63, 88  m m |
| NGC6826 |
PN |
52, 57, 63, 88  m m |
| NGC7027 |
PN |
52, 63, 88, 145, 158  m m |
| G298.228 |
HII region |
52, 57, 63, 88, 145, 158  m m |
| IRAS15408-5356 |
HII region |
52, 57, 63, 88, 145, 158  m m |
| NGC6302 |
HII region |
52, 57, 63, 88, 122, 145, 158  m m |
| IRAS23133 |
HII region |
63, 145, 158  m m |
| FP wavelength calibration |
| NGC7027 |
PN |
63.184 [OI], 157.741 [CII] |
| NGC3603 P1 |
HII region |
51.814 [OIII], 57.317 [NIII], 157.741 [CII] |
| NGC6826 |
PN |
51.814 [OIII], 88.356 [OIII], 157.741 [CII] |
| G0.6-0.6 |
HII region |
51.814 [OIII], 57.317 [NIII], 63.184 [OI], 121.898 [NII] |
| |
|
145.525 [OI], 157.741 [CII] |
|
The accuracy of the grating wavelength calibration has been checked by
measuring the central wavelengths of the lines observed in a large number
of auto analysis results from observations of NGC7027, NGC6543, S106
and W Hya. This check has shown that the wavelength calibration is
better than 1/4 of a resolution element of the grating (i.e. 0.068  m
or about 330 km/s at 60
m
or about 330 km/s at 60  m, and 0.145
m, and 0.145  m or about 290 km/s at
150
m or about 290 km/s at
150  m).
The width of the resolution element was also determined from these
observations. For the SW detectors it was determined to be 0.27
m).
The width of the resolution element was also determined from these
observations. For the SW detectors it was determined to be 0.27  m
and for the LW detectors 0.58
m
and for the LW detectors 0.58  m. (The nominal values derived
from ground based tests were 0.29
m. (The nominal values derived
from ground based tests were 0.29  m and 0.60
m and 0.60  m respectively.)
m respectively.)
The accuracy that is achieved for the wavelength with the grating is given
in Table 5.4.
Table 5.4:
Accuracy of
the wavelengths for an LWS spectrum. These accuracies are based on
actual measurements. 85% of all measurements were within these limits
for the accuracy. Note that the accuracy for the FPs can be improved
using the new calibration coefficients given in section 7.7.
| mode |
Accuracy |
| grating calibration |
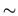 25% of a resol. element 25% of a resol. element |
| |
0.068  m for SW detectors m for SW detectors |
| |
0.145  m for LW detectors m for LW detectors |
| FPS calibration |
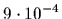  m RMS m RMS |
| |
scatter in the fitted calibration |
| FPL calibration |
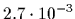  m RMS m RMS |
| |
scatter in the fitted calibration |
| FP absolute accuracy |
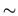 10 km/s or 1/3 of 10 km/s or 1/3 of |
| |
a resolution element |
|
The wavelength calibration of the LWs Fabry-Perots was derived using
observations of 4 sources with known velocities which show strong lines that
span a sufficient range in FP gap and wavelength values to adequately sample
the parameter space. The cetroid of the measured response is taken to
corerspond to the line center (since lines are clearly not gaussian in
shape, a gaussian fit to the lines can not be used). The sources and lines
that were used are given in Table 5.3.
The internal accuracy of the calibration is given by the RMS noise on
the polynomial fits done to obtain the calibration coefficients . This was
determined to be
 m for FPS and
m for FPS and
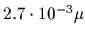 m for FPL.
m for FPL.
Another check for the accuracy of the FP wavelength calibration was done
with the weekly calibration monitoring observations. A total of 7 lines
(3 on FPS and 4 on FPL) in 7 sources was observed regularly with an L04 AOT
during the calibration time. The sources and lines that were used are
listed in Table 5.5.
Table 5.5:
The sources
and lines in this table were used for the monitoring of the FP wavelength
calibration.
| Source |
Lines |
| G0.6-0.6 |
51 ([OIII]), 57 ([NIII]), 88 ([OIII]), 122 ([NII]), 157  m ([CII]) m ([CII]) |
| G36.3-0.7 |
51 ([OIII]), 88  m ([OIII]) m ([OIII]) |
| NGC3603 |
57 ([NIII]), 88  m ([OIII]) m ([OIII]) |
| NGC6302 |
57 ([NIII]), 157  m ([CII]) m ([CII]) |
| NGC7023 |
63 ([OI]), 145 ([OI]), 157  m ([CII]) m ([CII]) |
| NGC7027 |
51 ([OIII]), 63 ([OI]), 145 ([OI]), 157  m ([CII]) m ([CII]) |
| NGC7538 |
51 ([OIII]), 63 ([OI]), 88 ([OIII]), 157  m ([CII]) m ([CII]) |
| S106 |
63 ([OI]), 157  m ([CII]) m ([CII]) |
|
These monitoring observations have been used to determine RMS errors
for the determination of the wavelengths with the FPS and FPL. These
errors are given in Table 5.6. The errors are for almost all
lines smaller than 10 km/sec. It should be noted however, that for the FPL,
these errors tend to be systematic the error gets bigger as the gap between
the FP etalons gets bigger (the 157 micron line is at the smallest
gap value, the 145 micron line at the largest). This points to a small error
in the FPL calibration, especially in the higher order coefficients, that are
not well determined.
Table 5.6:
The RMS errors in the
wavelengths determined from the FP monitoring observations.
| Line |
RMS error |
| |
 m m |
km/sec |
| FPS |
| [OIII] 51.8 |
0.95 |
5.5 |
| [NIII] 57.3 |
0.72 |
3.8 |
| [OI] 63.2 |
0.87 |
4.1 |
| FPL |
| [OIII] 88.4 |
1.5 |
5.1 |
| [NII] 121.9 |
2.2 |
5.4 |
| [OI] 145.5 |
6.6 |
13.6 |
| [CII] 157.7 |
3.0 |
5.6 |
|
Independently the accuracy with which the wavelength of a line can be
determined was checked using a set of observations of a number of objects.
For NGC7023 the central wavelengths of three lines (63, 145, 157  m)
were determined for a number of repeated observations. The velocities
derived from repeated observations of the same line are equal to within
10 km/sec, showing that the accuracy of the calibration with respect to
repeated observations of the same source is better than 10 km/sec.
m)
were determined for a number of repeated observations. The velocities
derived from repeated observations of the same line are equal to within
10 km/sec, showing that the accuracy of the calibration with respect to
repeated observations of the same source is better than 10 km/sec.
Further tests, using observations of sources with known velocities, show that
the velocities derived from the LWS FP observations are within 10 km/sec
from the values quoted in the literature. Also the difference between
velocities determined with FPS and FPL is smaller than 10 km/sec. We thus
conclude that the accuracy of the LWS Fabry Perot wavelength calibration is
10 km/sec, or one third of the LWs spectral resolution element.
The accuracy that is achieved for the wavelength with the FPs is given
in Table 5.4.
5.4.2 Photometric calibration
The accuracy of the photometric calibration is determined by a number of
factors:
- The accuracy of the model for Uranus that was used to calculate the
absolute response is believed to be about 5% for most detectors.
For the SW1 detector the model may be wrong. In addition some spectral
features that are present in the spectrum of Uranus were not present
in the model, leading to spurious features in the spectra (see
section 7.6).
- The measurement of Uranus that was used for the calibration has
a high S/N. However, for sources brighter than Uranus, that are
observed long enough to theoretically give a S/N higher than obtained
for the Uranus spectrum, the S/N of the resulting spectrum is limited by
the uncertainty in the RSRF. The uncertainty in the RSRF is written
in the Calibration file containing it (see section 8.2.9.4).
- Transients or memory effects may have an influence on the photometric
accuracy of the data. The extend of their influence is not clear at this
time.
- Ramp (non) linearity will also influence the accuracy. It is believed
that Derive-SPD is handling this reasonably well, but improvements are
being investigated. The size of this effect is not clear at this time, and
will depend also on the source (see section 7.3).
- The dark background removal will, especially for faint sources be
an important factor in the photometric accuracy. The size of this effect
depends on the source strength and the spectral shape (see
section 7.15). For very faint
sources the drift correction applied in AAL may result in negative
fluxes (see section 7.13).
- Glitches also influence the photometric accuracy, since they have an
effect on the responsivity of the detectors. The size of this effect
depends on the time in the orbit when the observation was carried out.
All these factors together lead to a photometric accuracy for LWS grating
mode spectra of 10% between repeated scans on the same detector (this is
mainly due to the effect of responsivity changes), and 30% between
adjacent detectors (mainly due dark background removal problems for faint
point sources and to source extend for extended sources).
For the Fabry-Perot mode, the photometric accuracy was determined by
comparing the integrated line fluxes observed with the FP with the fluxes
observed with the grating or line fluxes published in the literature. The
sources and lines are given in Table 5.7. It was found that
for strong lines accuracy is typically better than 30%. For faint lines
however, the FP fluxes can be off by almost a factor 2. This is mainly due
to the removal of the darkcurrent which is known to be problematic
for low signal levels (see also section 7.13 and 7.15).
Table 5.7:
The sources
and lines in this table were used for the determination of the photometric
accuracy of the Fabry-Perot data.
| Source |
Type |
Lines |
| NGC6543 |
PN |
57, 88  m m |
| NGC7027 |
PN |
51, 63, 145, 157  m m |
| NGC6357I |
HII region |
52, 57, 63, 88, 145, 157  m m |
| M82 |
Galaxy |
63, 88, 122, 157  m m |
|




Next: 6. Data processing
Up: ISOLWS DATA USERS MANUAL
Previous: 4. Instrument Characteristics
ISOLWS Data Users Manual, Issue 5.0, SAI/95-219/Dc
![]() m
or about 330 km/s at 60
m
or about 330 km/s at 60 ![]() m, and 0.145
m, and 0.145 ![]() m or about 290 km/s at
150
m or about 290 km/s at
150 ![]() m).
The width of the resolution element was also determined from these
observations. For the SW detectors it was determined to be 0.27
m).
The width of the resolution element was also determined from these
observations. For the SW detectors it was determined to be 0.27 ![]() m
and for the LW detectors 0.58
m
and for the LW detectors 0.58 ![]() m. (The nominal values derived
from ground based tests were 0.29
m. (The nominal values derived
from ground based tests were 0.29 ![]() m and 0.60
m and 0.60 ![]() m respectively.)
m respectively.)
![]() m for FPS and
m for FPS and
![]() m for FPL.
m for FPL.
![]() m)
were determined for a number of repeated observations. The velocities
derived from repeated observations of the same line are equal to within
10 km/sec, showing that the accuracy of the calibration with respect to
repeated observations of the same source is better than 10 km/sec.
m)
were determined for a number of repeated observations. The velocities
derived from repeated observations of the same line are equal to within
10 km/sec, showing that the accuracy of the calibration with respect to
repeated observations of the same source is better than 10 km/sec.