|

|
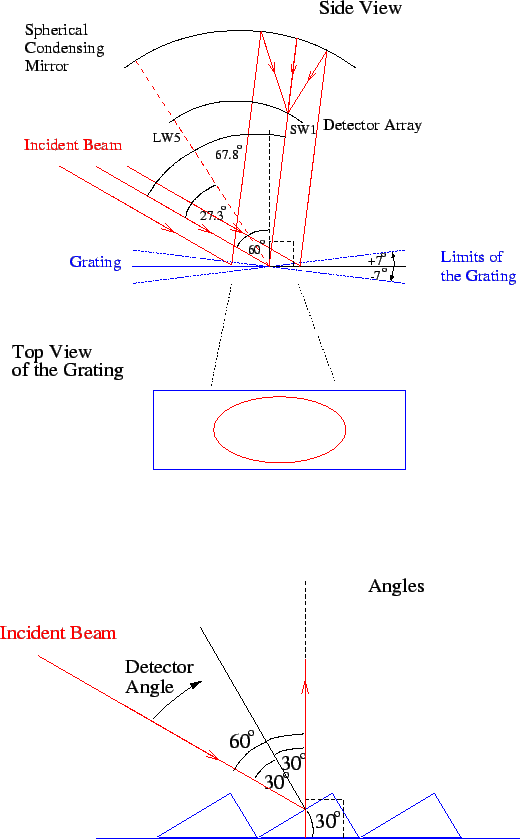
In its rest position, the grating normal was at 60![]() to the
incident beam (see Figure 2.4).
By rotating the grating, by means of a servo-controlled drive mechanism,
between
to the
incident beam (see Figure 2.4).
By rotating the grating, by means of a servo-controlled drive mechanism,
between ![]() and
and ![]() (the
physical limits of its motion), the centre of the grating response
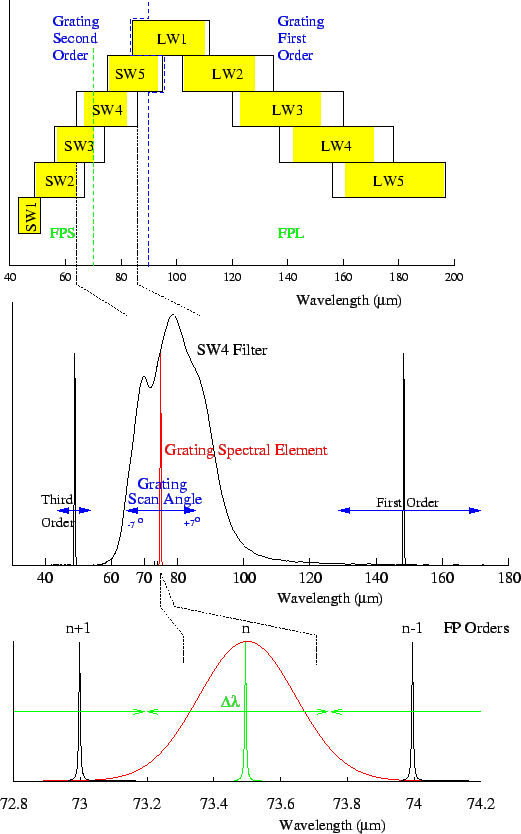
function was scanned over a wavelength band, for SW4 this ranged from 64 to
86
(the
physical limits of its motion), the centre of the grating response
function was scanned over a wavelength band, for SW4 this ranged from 64 to
86![]() m; it is shown in the upper panel of
Figure 2.5. Considering each detector in turn, a contiguous
coverage from 45 to 180
m; it is shown in the upper panel of
Figure 2.5. Considering each detector in turn, a contiguous
coverage from 45 to 180![]() m was achieved by rotating the grating from
m was achieved by rotating the grating from
![]() to
to ![]() . By using the extended range of
operation (
. By using the extended range of
operation (![]() to
to ![]() ) -- at the cost of a small
increase in power dissipated in the focal plane -- the spectral range was
extended to cover 43-197
) -- at the cost of a small
increase in power dissipated in the focal plane -- the spectral range was
extended to cover 43-197![]() m, whilst giving significant overlap between
the spectral coverage of adjacent-wavelength detector channels. In order to
maximise the sensitivity of the instrument at all wavelengths, the
extended scanning range has been used in normal operation.
The wavelength
ranges used for each detector, along with the overlaps, are indicated
in the upper panel of Figure 2.5 (note that
the limitations
on the detector wavelength ranges, as discussed below, are taken into
account). The nominal well calibrated ranges are
shown in yellow. The extended range gave
important verification when looking for weak line features and
afforded redundancy in the instrument if there had been a catastrophic
failure in one detector.
m, whilst giving significant overlap between
the spectral coverage of adjacent-wavelength detector channels. In order to
maximise the sensitivity of the instrument at all wavelengths, the
extended scanning range has been used in normal operation.
The wavelength
ranges used for each detector, along with the overlaps, are indicated
in the upper panel of Figure 2.5 (note that
the limitations
on the detector wavelength ranges, as discussed below, are taken into
account). The nominal well calibrated ranges are
shown in yellow. The extended range gave
important verification when looking for weak line features and
afforded redundancy in the instrument if there had been a catastrophic
failure in one detector.
|
The grating diffracts radiation of
wavelength ![]() in first order at the same
angle as wavelength
in first order at the same
angle as wavelength ![]() in second order and as wavelength
in second order and as wavelength
![]() in
in ![]() order. With this constraint it
was not possible to utilise the full wavelength coverage afforded by
the range of possible scan angles. To ensure that only the required
narrow band of wavelengths is detected at a particular grating angle,
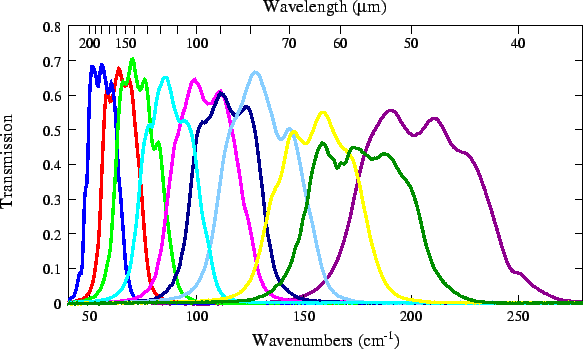
and not the wavelengths in different orders, filters with well-defined
passbands were placed in front of the
detectors. The transmission of the filters, measured by Ade
(private communication), is shown in
Figure 2.6. The resulting nominal wavelength
limits for each band are given in Table 2.3.
They take into account the following limitations:
order. With this constraint it
was not possible to utilise the full wavelength coverage afforded by
the range of possible scan angles. To ensure that only the required
narrow band of wavelengths is detected at a particular grating angle,
and not the wavelengths in different orders, filters with well-defined
passbands were placed in front of the
detectors. The transmission of the filters, measured by Ade
(private communication), is shown in
Figure 2.6. The resulting nominal wavelength
limits for each band are given in Table 2.3.
They take into account the following limitations:
|
|
The central panel in Figure 2.5 shows the nominal
wavelength
range covered by SW4, as determined by its filter. When the grating was in
its rest position, the central wavelength
falling on this detector was 75![]() m. With the grating at an angle of
m. With the grating at an angle of
![]() its spectral response
function (red) fell at 73.5
its spectral response
function (red) fell at 73.5![]() m. The lower panel shows the
range of the wavelengths that fell on the detector at this angle, as
given by the grating spectral element (red).
m. The lower panel shows the
range of the wavelengths that fell on the detector at this angle, as
given by the grating spectral element (red).
| Det | Y | X | Detector | Bandpass | Edge Filter | ||
| Measured | Design | Measured | Design | Serial | Filter Serial | Serial | |
| [ |
[ |
[mm] | [mm] | Number | Number | Number | |
| SW1 | 494 | 500 | 1.34 | 1.29 | 7 | 325 | 8 |
| SW2 | 529 | 530 | 1.32 | 1.29 | 9 | 321 | 50 |
| SW3 | 529 | 540 | 1.30 | 1.29 | 13 | 319 | 6 |
| SW4 | 617 | 610 | 1.34 | 1.29 | 11 | 313 | 12 |
| SW5 | 640 | 650 | 1.35 | 1.29 | 12 | 309 | 9 |
| LW1 | 580 | 570 | 1.34 | 1.29 | 10 | 306 | 2 |
| LW2 | 617 | 620 | 1.35 | 1.29 | 55 | 302 | 1 |
| LW3 | 652 | 650 | 1.34 | 1.29 | 56 | 328 | 5 |
| LW4 | 700 | 690 | 1.32 | 1.29 | 58 | 296 | 4 |
| LW5 | 758 | 750 | 1.30 | 1.29 | 57 | 292 | 3 |
The re-imaged size of the beam, ![]() , at the detector array was
determined by the focal ratios of the collimator (
, at the detector array was
determined by the focal ratios of the collimator (![]() ) and
the condenser (
) and
the condenser (![]() in the dispersion direction and
in the dispersion direction and
![]() in the non-dispersion direction as dictated by the
anamorphic magnification, see below) and the size of the
focal plane aperture, M2 (
in the non-dispersion direction as dictated by the
anamorphic magnification, see below) and the size of the
focal plane aperture, M2 (![]() ) such that:
) such that:
Equation 2.1 gives the aperture size in the dispersion direction to be 0.465mm. However, there is a modification of the beam cross-section which is referred to as anamorphic magnification (AMAG; the ratio of the diameter in the non-dispersion direction to that in the dispersion direction). It occurred because the radiation was not specularly reflected with respect to the plane of the grating, hence the emergent beam was elliptical. (The incident beam was circular and it made an elliptical footprint on the grating, but this was due to purely geometrical effects.) This AMAG reduced the image size such that a detector aperture of 0.7mm was actually required for the dispersion direction.
AMAG was smallest for the most positive scan angles (long wavelength end) of each detector range, so to ensure good efficiency the positive scan angle limit was used to determine the beam size and consequently the aperture size for each detector in the dispersion direction. The final measured and designed aperture sizes for the detectors are given in Table 2.1 along with the serial numbers of the actual detectors, bandpass filters and edge filters that flew on ISO.
For the LWS the AMAG is typically equal to
two. As the parallel beam had a width of 34mm, the
beam was typically dispersed over 68mm. The
grating was ruled with 7.9 lines per mm, hence the number of lines
covered by the beam in the dispersion direction was ![]() 540. For the
above configuration,
the chromatic resolving power (
540. For the
above configuration,
the chromatic resolving power (![]() , where
, where ![]() is the order and
is the order and ![]() is
the number of lines) is
is
the number of lines) is ![]() 1080 in second order and
1080 in second order and ![]() 540 in
first.
However, in reality the chromatic resolution depends on the detector
location (as different detectors view the grating at different angles) and
also on the scan angle of the
grating.
The LWS beam size is wavelength dependent. An effective aperture
radius for each detector has been defined by Lloyd 2000, [27]
(see Section 5.9)
and is listed in Table 5.9.
540 in
first.
However, in reality the chromatic resolution depends on the detector
location (as different detectors view the grating at different angles) and
also on the scan angle of the
grating.
The LWS beam size is wavelength dependent. An effective aperture
radius for each detector has been defined by Lloyd 2000, [27]
(see Section 5.9)
and is listed in Table 5.9.
This array was therefore capable of simultaneously detecting ten
spectral elements within the LWS spectral region. However, the packing
density of the detectors was sparse (limited by the size of the
detector mounts), so to get complete spectral coverage the grating had
to be scanned to move the wavelengths sequentially across each
detector.
By having ten detectors rather than one, the whole spectrum could be
obtained in a tenth of the time. Because of the wide spectral coverage
of the LWS, it was necessary to use the grating in first order for
wavelengths from 94.6-196.9![]() m and second order for wavelengths
43-94.6
m and second order for wavelengths
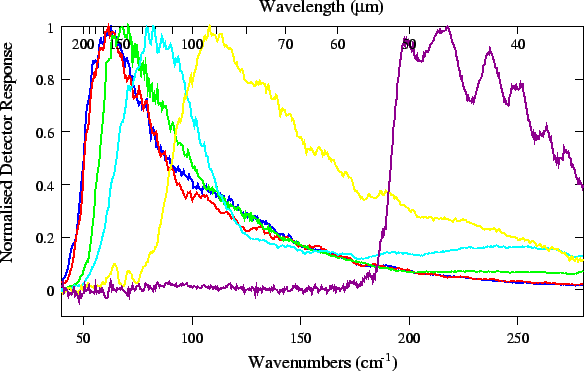
43-94.6![]() m, to maximise its efficiency. The grating efficiency
measurements, as performed by Petti 1989, [31], are shown in
Figure 2.8. Because of the two orders used in the LWS,
it was necessary to interleave the long wavelength detectors between
the short wavelength detectors to make optimum use of the limited
space available whilst maintaining the maximum spectral
range. Accordingly, the detectors are labelled SW1 though SW5 for the
short wavelength set and LW1 to LW5 for the long wavelength set. The
detectors in their different positions saw the grating at different
angles. The diffracted beam for detector SW1 emerged at
an angle of
m, to maximise its efficiency. The grating efficiency
measurements, as performed by Petti 1989, [31], are shown in
Figure 2.8. Because of the two orders used in the LWS,
it was necessary to interleave the long wavelength detectors between
the short wavelength detectors to make optimum use of the limited
space available whilst maintaining the maximum spectral
range. Accordingly, the detectors are labelled SW1 though SW5 for the
short wavelength set and LW1 to LW5 for the long wavelength set. The
detectors in their different positions saw the grating at different
angles. The diffracted beam for detector SW1 emerged at
an angle of ![]() with respect to the grating normal, as
shown in the top part of Figure 2.4. A
simplistic way to determine where the detectors were located in the
LWS is to refer to the angle between the incident beam and the direction
of the detector from the grating, as tabulated in
Table 2.2.
with respect to the grating normal, as
shown in the top part of Figure 2.4. A
simplistic way to determine where the detectors were located in the
LWS is to refer to the angle between the incident beam and the direction
of the detector from the grating, as tabulated in
Table 2.2.
| Detector | Detector Angles [ |
Wavelength [ |
||
| Ground | In Orbit | Ground | In Orbit | |
| SW1 | 67.938 | 67.80 | 46.0711 | 46.2220 |
| LW1 | 63.411 | 63.26 | 102.092 | 102.425 |
| SW2 | 58.889 | 58.74 | 56.0389 | 56.2033 |
| LW2 | 54.370 | 54.29 | 122.042 | 122.218 |
| SW3 | 49.885 | 49.71 | 65.9272 | 66.1173 |
| LW3 | 45.340 | 45.27 | 141.659 | 141.809 |
| SW4 | 40.825 | 40.73 | 75.6000 | 75.6989 |
| LW4 | 36.308 | 36.275 | 160.487 | 160.554 |
| SW5 | 31.785 | 31.72 | 84.7346 | 84.7977 |
| LW5 | 27.256 | 27.32 | 178.090 | 177.971 |